Several TOD commenters have asked for a simple and intuitive definition for how the shock model works, and it has always been a challenge to express it concisely. In mathematical terms, it is simply the application of the convolution function to a model of the statistical flow rate operating on the reserve potential of the reservoirs of interest.

The problem in casting it in this stark a mathematical form has been that the concept of convolution is neither intuitive nor readily available to the layman. For example, the Excel spreadsheet application does not have a convolution function in its toolbox of statistical operators. This is odd considering that the great statistician William Feller once remarked that “It is difficult to exaggerate the importance of convolutions in many branches of mathematics.”

The best intuitive explanation that I can come up with is that a convolution (in the oil production context) is a "sliding" summation of extraction applied to reserves.
Thus, the convolution algorithm automatically keeps track of older reserves as well as new reserves as the total production accumulates with varying levels of extraction over time. Whether this is completely intuitive to the layperson, we can always remember that a convolution is largely a cookbook accounting exercise and once the form of the two inputs are known, a simple algorithm can be applied to obtain a result.

For modeling the Bakken ala the convolution-based shock model, the inputs are two time-series.

1. The forced input is the time series of newly available wells.
2. The response input is the time series of expected decline from a single well.
   The convolution function takes the forced input and applies the response input and generates the expected aggregate oil production over time.

DC at his blog http://OilPeakClimate.blogspot.com/ has used this approach to good effect in modeling historical and projecting future Bakken production. I apply a slightly different response function than DC and get this shock model output:

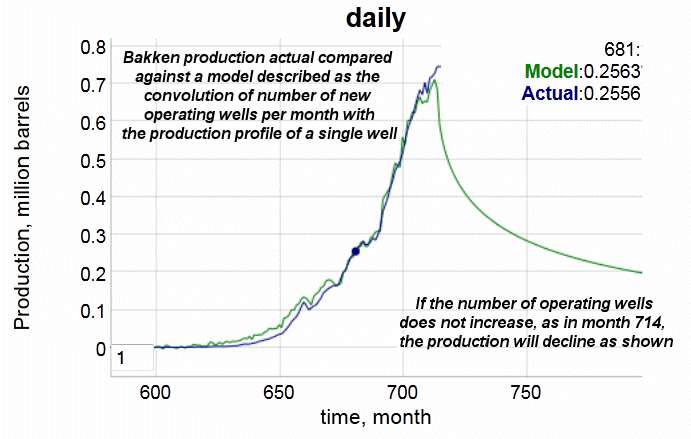
Month #714 on the time series is essentially up-to-date, so that this is a modeled profile of the past 60 years of Bakken activity, using the historical monthly well numbers as input (from http://www.dmr.nd.gov/oilgas/stats/historicalbakkenoilstats.pdf).

The two curves correspond to (1) the actual production data and (2) that which is modeled after applying the convolution-based shock model to the well build-up, assuming a fairly rapid decline response per well. The decline after month 714 would show what would happen if no new wells were added. That of course won't happen, but it illustrates the Red Queen effect that Rune Likvern has argued on these pages. The Red Queen hypothesis is that production will continue to increase as long as a fresh supply of new wells with nominal reserve potential comes on line at a good pace.

As a detail, where DC and I differ is in how we apply the response model for the average well. I have been applying a diffusional model based on the physics of flow, whereas DC has been using a hyperbolic decline model which is favored by reservoir engineers. Not much of a difference between the two, apart from gaining an understanding of what is actually happening underground, which is likely an initially rapid diffusional flow followed by a the long tails of a diffusional decline.

As a caveat, the model would likely work even better if the North Dakota Department of Mineral Resources had kept a cumulative total instead of an active count in their PDF table --
but as is the case with most of the data, you use what you can get.

The take-home point is that analysis approaches do exist outside of the insider oil patch knowledge-base. Us mere mortals can formulate and apply these simple models to at least try to get a handle on future fossil fuel supplies. That was the objective that I had when I started my blog and followed along with TOD as we watched crude oil production plateau the last 9 years.

---

Doing this work on applying probability and statistics to the energy predicament has opened up other possibilities which I have since pursued. Recently I have started up another blog on general environmental modeling called http://ContextEarth.com. This has an associated interactive modeling web server called the Dynamic Context Server, which builds up from a semantically-organized knowledge-base of land, water, and atmospheric information.

I have incorporated the shock model as one of the functionalities in the server and intend to maintain other capabilities to make it useful for environmental model activities, such as wind, solar, and transportation simulations. Comments and collaboration opportunities are welcomed.

As you can see, The Oil Drum is only a start to the on-going energy transformation that we are going through.